oramapax.blogg.se - Trig graphs cheat sheet

Keep it handy while you’re revising the concept, especially before an exam. This one page PDF covers summarised theory and the most important formulas related to the concept. Cheat sheets on Graph Transformations ( Basic and Advanced) are also available on this website. This handout includes instructions for graphing processes of basic, amplitude shifts, horizontal shifts, and vertical shifts of trigonometric functions. You also must have a strong grasp on graph transformations, which will help you to understand the relation between the graphs of trigonometric functions and inverse trionometric functions. Cheat sheets on Trigonometry and Inverse Trigonometric Functions are also available on this website. A study of these functions gives a good insight into the behaviour, domains and ranges of inverse functions in general.Ī good knowledge of the basic formulae of trigonometry and inverse trigonometric functions is a must to understand and solve problems related to inverse trigonometry. This topic is a part of trigonometry and is an intersection of two major concepts – inverse of a function and trigonometry. Actually, this only works if x is positive. Using the area formula for a sector (from the previous page) and the area formula for a triangle (from heart), this becomes 1 2 ·cos2(x)·x 1 2 ♱·sin(x) 1 2 ♱2 ·x.

One must look for the minimum as well as the maximum values of y.Īnswer: A function refers to an expression that shows the relationship between the one variable and another variable.This cheat sheet covers the high school math concept – Inverse Trigonometric Functions Graphs. 150 Limits of Trigonometric Functions Area of sector OAB Area of triangle OCP Area of sector OCP.

Substitution of different x-values into the expression for y so as to understand what is going on.

The range of a function happens to the spread of possible y-values.

Question 4: How can one find the range of a function?Īnswer: One can find the range of a function by the following steps: Usually one must avoid 0 on the fraction’s bottom and the negative values which are under the square root sign. Question 3: How can one find the domain of a function?Īnswer: One can determine the domain of each function by looking for independent variables values which one is allowed to use. The range means the set of possible output values whose representation takes place on the y-axis.

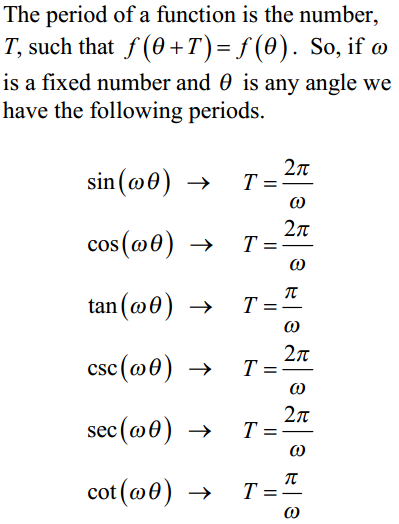
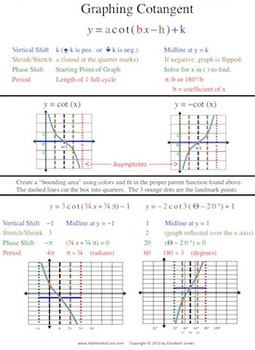
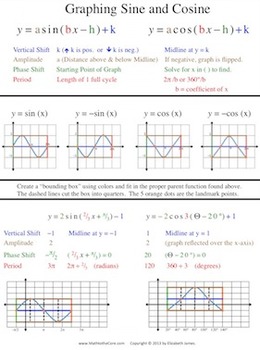
The domain of a graph involves all the input values which are represented on the x-axis. General Form of Sine and Cosine Funtions (on cheat sheet). Question 2: What is meant by domain and range?Īnswer: Domain means the set of possible input values. Since the cosine graph is a horizontal translation of the sine graph it is a sinusoidal function. We also know that for each real number ‘x’, We know that the sine and cosine functions are defined for all real numbers. Domain and Range of Trigonometric Functions